This post gives a derivation of the Black-Litterman (BL) model. BL model is a bespoke tactical asset allocation model incorporating investor’s view for tilting market equilibrium weights. The BL posterior expected returns can be obtained by using the Theil mixed estimator.
Black-Litterman Model
Goldman Sachs says that “Fischer Black and Robert Litterman revolutionize portfolio management in 1990 with the creation of the Black-Litterman Global Asset Allocation Model – quickly adopted for optimal portfolio allocation across international equity, fixed income and currency markets”.
Black-Litterman (BL) model uses the Theil mixed estimator which is a kind of GLS estimator. You can find more information regarding these estimators in the following previous posts.
Prior Expected Returns
Prior expected returns relies on market equilibrium returns or market clearing returns. To find these returns, a reverse optimization is used.

where
- Π : the implied excess equilibrium return vector (N × 1)
- λ : the risk aversion coefficient (scalar)
- Σ : the covariance matrix of excess returns (N × N)
- wm : the market capitalization weight of assets (N × 1)
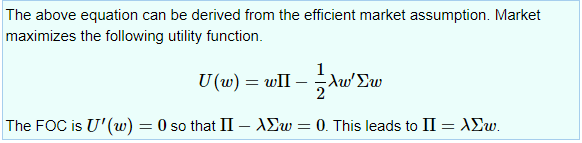
Uncertainty of Prior Expected Returns
“Expected” is always followed by “uncertainty” or error. While the market clearing returns (Π) are “estimates” and are known, the true expected returns (μ) are unknown. This leads to the following equation.
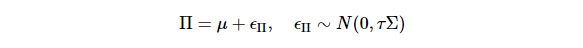
for some small scale factor parameter τ≪1. A small τ means a high confidence in equilibrium estimates. This reflects that the variance in the expected returns is smaller than the variance of the actual returns.
Investor’s Views
Views of BL model can be relative or absolute. K views are expressed as K linear combinations of the true excess return vector (μ). It is a common practice to set the off diagonal elements of Ω to zero due to the independence assumption for each views.
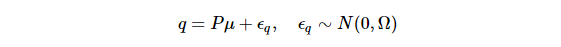
where P is a K × K pick matrix and Ω is a K × K matrix of confidence in the views.
Matrix Representation
Let’s collect two equations and represent it as a matrix form.
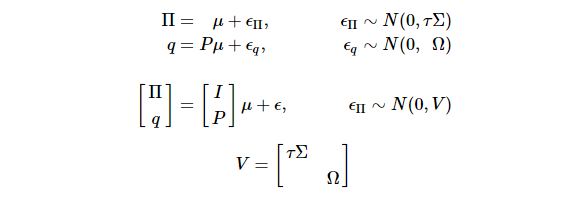
where I is the N × N identity matrix. We are familiar with this matrix representation.
The Posterior Expected Returns
Using Theil mixed estimator (GLS estimator) which is explained in the previous posts, we can get the following posterior returns (μBL).
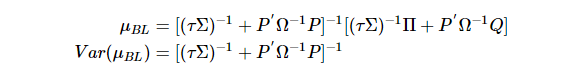
This is a combined expected returns of the market equilibrium and investor’s views.
Concluding Remarks
This post delivers the derivation of the famous the Black-Litterman model using the Theil mixed estimator. For a clear understanding of BL model, I also explained the GLS estimator and Theil mixed estimator in the earlier posts. Real applications will be treated in the next post
Originally posted on SHLee AI Financial Model blog.
Disclosure: Interactive Brokers Third Party
Information posted on IBKR Campus that is provided by third-parties does NOT constitute a recommendation that you should contract for the services of that third party. Third-party participants who contribute to IBKR Campus are independent of Interactive Brokers and Interactive Brokers does not make any representations or warranties concerning the services offered, their past or future performance, or the accuracy of the information provided by the third party. Past performance is no guarantee of future results.
This material is from SHLee AI Financial Model and is being posted with its permission. The views expressed in this material are solely those of the author and/or SHLee AI Financial Model and Interactive Brokers is not endorsing or recommending any investment or trading discussed in the material. This material is not and should not be construed as an offer to buy or sell any security. It should not be construed as research or investment advice or a recommendation to buy, sell or hold any security or commodity. This material does not and is not intended to take into account the particular financial conditions, investment objectives or requirements of individual customers. Before acting on this material, you should consider whether it is suitable for your particular circumstances and, as necessary, seek professional advice.













Join The Conversation
For specific platform feedback and suggestions, please submit it directly to our team using these instructions.
If you have an account-specific question or concern, please reach out to Client Services.
We encourage you to look through our FAQs before posting. Your question may already be covered!